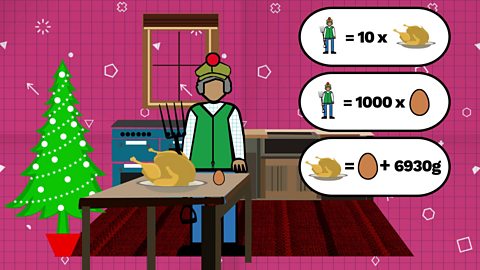
Problem 5 - Powerful pyramid
Problem 5 is about powers of 2, like 2² or 2³.
Chris Smith is here to help you square up to this challenge.
The Maths Week Scotland Daily Challenges have been set by the Scottish Mathematical Council.
Chris Smith: Today’s problem is all about the number 2.
2 is a number with some very special powers.
Let me show you what I mean.
In maths, when we talk about powers, we mean how many times 2 has been multiplied by itself.
So 2 to the power of 1 (that’s the little raised up 1) is just 2.
So 2 to the power of 2 (or 2 squared) equals 2 x 2, which equals 4.
2 to the power of 3 (or 2 cubed) is 2 x 2 x 2, which equals 8.
2 to the power of 4 equals 2 x 2 x 2 x 2, which equals 16.
We can keep building up the powers until we form a Pyramid of Powers!
Now look at the final digits of all the answers in the pyramid of powers.
Can you work out the final digits of 2 to the power of 8, 2 to the power of 9, 2 to the power of 20 and 2 to the power of 111.
For the first few answer you can write out all the twos and multiply them…but you’d need a lot of paper to do that for 2 to the power 111.
Did you see a pattern in the final digits of our powerful pyramid? There lies the key to this problem!
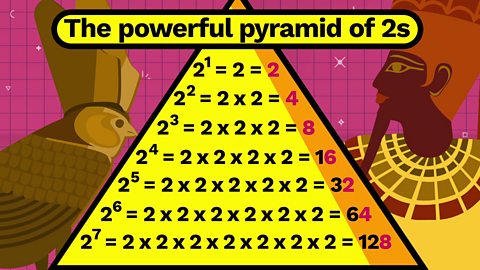
On the left of the pyramid we have what are called the powers of 2.
The power itself (sometimes called an index) is the little number that is raised slightly. It tells us how many 2s we multiply together.
- 2¹ just means 2
- 2² means 2 x 2 which equals 4
- 2³ means 2 x 2 x 2 which equals 8
On the right of the pyramid are the values of each of the powers of two.Look at the final digits of these values and answer this four-part question:
What is the final digit of:
- a) 2⁸
- b) 2⁹
- c) 2²⁰
- d) 2¹¹¹
Explain how you obtained your answers?

Need a hint?
For the first few answer you can write out all the twos and multiply them…but you’d need a lot of paper to do that for 2 to the power 111.
See if you can spot a pattern in the final digits of our powerful pyramid? That's the key to solving the larger powers.
Solution
Worked out the answer? Here's how you can do it.
Chris Smith: How did you get on with the pyramid problem? Did you spot a pattern that helped you to solve this one?
A wee reminder: I was looking for you to find the final digits of 2⁸, 2⁹, 2²⁰, 2¹¹¹.
Let’s take a look at our powerful pyramid again.
It looks like the final digits in the pyramid repeat the pattern 2, 4, 8, 6. And we suspect that this will keep repeating forever.
We can work out the first two answers to our problem by calculating the next two lines of the pyramid. You just double the number each time.
2⁸ = 128 x 2 = 256.
And 2⁹ = 256 x 2 = 512.
So the answers to our first two questions are 2 to the power 8 ends in 6 and 2 to the power 9 ends in 2.
And we’ve confirmed that the 2, 4, 8, 6 pattern is continuing.
So to find the answer to the third value - 2²⁰ - just continue the 2, 4, 8, 6 pattern. You don’t even need to do the calculations – just note down the sequence of final numbers in ten rows till you get to 2²⁰ and you can see that its final number is a 6.
Even if we're just writing down the final digit in the powerful pyramid It’s still going to take a lot of paper to get all the way down to 2¹¹¹.
But there’s another pattern hiding in this problem. Did you see it?
All of the powers of 2 that are multiples of 4 - 2⁴, 2⁸, 2¹² and so on – they all end in the number 6.
How does this help us? Well, we need to find out about 2¹¹¹ , and 111 isn’t a multiple of 4. But 112 is a multiple of 4 so we know 2¹¹² end in a 6.
2¹¹¹ is the number before this, so we can use the 2, 4, 8 , 6 pattern to work out that 2¹¹¹ must end in an 8.
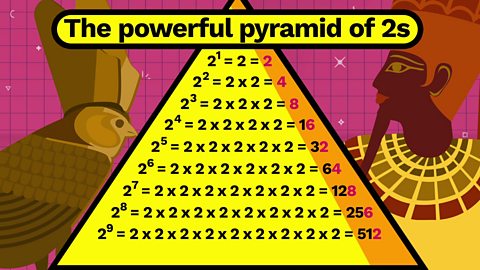
It looks like the final digits in the pyramid repeat the pattern 2, 4, 8, 6. And we suspect that this will keep repeating forever.
We can work out the first two answers to our problem by calculating the next two lines of the pyramid. You just double the number each time.
We had got down to 2⁷, which equals 128.
So 2⁸ = 128 x 2 = 256.
And 2⁹ = 256 x 2 = 512.
The answers to our first two questions are 2⁸ ends in 6 and 2⁹ ends in 2.


We can use the 2, 4, 8, 6 pattern to find the final digit of 2²⁰
We don’t need to do the calculations. The pattern means we can just note down the sequence of final numbers until we get to 2²⁰.
| power of two | final digit |
|---|---|
| 2¹⁰ | 4 |
| 2¹¹ | 8 |
| 2¹² | 6 |
| 2¹³ | 2 |
| 2¹⁴ | 4 |
| 2¹⁵ | 8 |
| 2¹⁶ | 6 |
| 2¹⁷ | 2 |
| 2¹⁸ | 4 |
| 2¹⁹ | 8 |
| 2²⁰ | 6 |
So 2²⁰ ends in a 6.

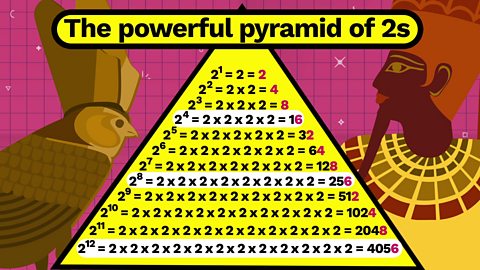
Writing down the final digits all the way down to 2¹¹¹ would be a long process and it would be easy to make a mistake.
There’s another pattern hiding in this problem that can get us to the answer more quickly.
All of the powers of 2 that are multiples of 4 - 2⁴, 2⁸, 2¹² and so on – end in the number 6.


2¹¹¹ doesn't have a power that is a multiple of 4. But 2¹¹² does because 112 is a multiple of 4. So 2¹¹² ends in a 6.
2¹¹¹ is the number before this, so we can use the 2, 4, 8 , 6 pattern to work out that 2¹¹¹ must end in an 8.

More on Problem solving
Find out more by working through a topic
- count20 of 21
- count21 of 21

- count1 of 21

- count2 of 21