Problem 5 - Dominoes
Problem 5 is all about finding all the possibilities for fitting a set of dominoes in a box.
Maths teacher Chris Smith and pupils from Grange Academy are here to explain.
The Maths Week Scotland Daily Challenges have been set by the Scottish Mathematical Council.
CHRIS This puzzle is all about dominoes and thinking outside… or inside the box.
Pat is tidying up after a game of dominoes.
Pat places four dominoes in a four by two box then does it again in a different way and then in a different way again.
After a little while, she realises that there are five ways it can be done. Here are the five ways Pat found.
Pat wonders what would happen if she had six dominoes and fitted them into a six by two box.
She managed to find all the possibilities.
How many are there? Can you find them?
And it's all about thinking about the number of ways that we can fit dominoes into a particular box.
PUPILS
You can draw these out or use some real dominoes.
Think of a domino as a rectangle that is twice as long as it is wide.
Remember the domino can be turned around to be placed in the box.
Good luck.
So here's the challenge:
Pat places four dominoes in a 4 x 2 box, then does it again in a different way, and then in adifferent way again. After a little while she realises that there are five ways it can be done.
Here are the five ways Pat found:
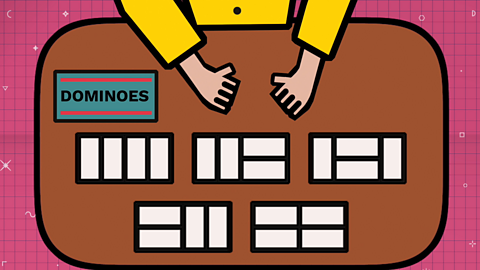

Pat wonders what would happen if she had six dominoes and fitted them into a 6 x 2 box.
She managed to find all the possibilities.
How many are there? Can you find them?

Need a hint?
You can draw the dominoes out or use some real dominoes.
Think of a domino as a rectangle that is twice as long as it is wide.
Remember the domino can be turned around to be placed in the box.
Solution
Worked out the answer? Here's how you can do it.
This challenge was all about dominoes and problem solving.
Pat wanted to know how she could fit six dominoes into a six by two box.
She manages to find all the possibilities.
How many possibilities were there?
The box is six dominoes long.
So you can have all six dominoes lined up in a row. There’s only one way of doing this.
Or you can turn two of them round. There are five different ways of doing this.
You can turn four of them round. There are six ways of doing this.
You can turn all six of them round. There’s just one way of doing this.
So in total there are one, plus five, plus six, plus one possibilities.
There were thirteen different possibilities in total.
Did you find them all?
If you enjoyed this challenge, here are some follow up questions for you to think about.
How many patterns for other numbers of dominoes?
What sequence of numbers is produced for one domino, two dominoes, three dominoes, four dominoes and so on?
How could binary digits be applied to the patterns?
Would this help to generate the answers?
In this challenge, Pat wanted to know how she could fit six dominoes into a 6 x 2 box.
Here are all the ways she could do this:
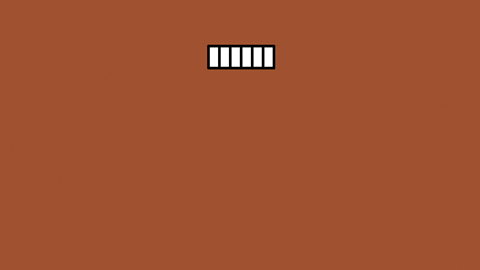
Image caption, Lined up in a row
The box is six dominoes long, so you can have all six dominoes lined up in a row. There is only one way to do this.

Image caption, Turn two round
You can keep four of the dominoes lined up and turn two dominoes round. There are five different ways of doing this.
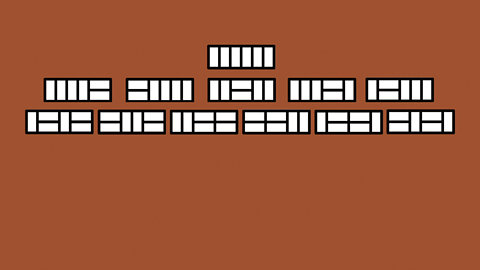
Image caption, Turn four round
You can keep two dominoes lined up and have four of them turned round. There are six ways of doing this.
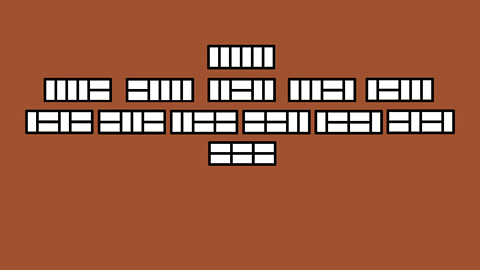
Image caption, Turn all six round
You can turn all six of them round. There’s just one way of doing this.
1 of 4

In total there are 1 + 5 + 6 + 1 possibilities.
There were 13 different possibilities in total.

Extension activity - Finding sequences
The more dominoes you use, the bigger the number of arrangements becomes. It can become quite tricky not to lose your place.Instead of counting each arrangement, it might be useful to look for a sequence that can help you predict the number of arrangements.
This table shows the number of arrangements for one, two, three, four, five and six dominoes.
| Number of dominoes | Number of arrangements |
|---|---|
| 1 | 1 |
| 2 | 2 |
| 3 | 3 |
| 4 | 5 |
| 5 | 8 |
| 6 | 13 |
| 7 | ? |
| 8 | ? |
Can you spot a sequence?
Can you predict how many arrangements there are for seven and eight dominoes?
Problem 5 - Dominoes
Have you noticed that as the number of dominoes increases, the number of arrangements can be found by adding the number of arrangements for the previous two numbers of dominoes?
Extension activity - Binary numbers
We normally use decimal numbers. These are based on the digit 0 - 9 and have place values of units, tens, hundreds etc.
Binary numbers are based on the digits 0 and 1 and have place values of units, twos, fours, eights, etc.
We can convert numbers between binary and denary.
The denary number 9 can be written in binary by splitting it into units, twos, fours and eights:
In binary, 9 is 1 x eight, 0 x four, 0 x two, 1 x unit, so we write it as 1001.
We can write the numbers of ways of arranging different numbers of dominoes in binary.
| Number of dominoes | 32s | 16s | 8s | 4s | 2s | 1s |
|---|---|---|---|---|---|---|
| 1 | 1 | |||||
| 2 | 1 | 0 | ||||
| 3 | 1 | 1 | ||||
| 4 | 1 | 0 | 1 | |||
| 5 | 1 | 0 | 0 | 0 | ||
| 6 | 1 | 1 | 0 | 1 | ||
| 7 | 1 | 0 | 1 | 0 | 1 | |
| 8 | 1 | 0 | 0 | 0 | 1 | 0 |
Does writing the numbers in binary make the pattern clearer for you?
Maths Week Scotland 2022. listMaths Week Scotland 2022
Try out all the daily challenges from Maths Week Scotland 2022.
Maths Week Scotland 2021. listMaths Week Scotland 2021
Want more maths challenges? Here are all the problems from 2021.
Maths Week Scotland 2020. listMaths Week Scotland 2020
Seven different maths problems from Maths Week Scotland 2020. Can you solve them all?
More on Problem solving
Find out more by working through a topic
- count13 of 21

- count14 of 21
- count15 of 21

- count16 of 21