Multiplication - partition method
In this method the smaller number is partitioned (broken down into tens and units).
Example
Use the partition method to work out \({352}\times{27}\).
Method 1
\({27}\) is broken down into \({20}\) and \({7}\).
Method 2
\({27}\) is broken down into \({10}\), \({10}\) and \({7}\).
The method of partitioning into tens, simplifies the multiplication further because to multiply successively by \({10}\) is to simply move the digits one place to the left.
Multiplication – Napier’s method
This method uses a grid to multiply numbers.
Multiplying a two-digit number with another two-digit number would require a \({2}\) by \({2}\) grid, for example \({43}\times{26}\).
Multiplying \({264}\times{53}\) would require a \({3}\) by \({2}\) grid.
Follow the steps below to see how Napier’s method is used to calculate \({43}\times{26}\).
- The first step is to draw a \({2}\) by \({2}\) grid.
- The second step is to draw a diagonal in each box. The diagonal line separates the tens and the units. Always write the tens above the diagonal line in each box.
- Start by multiplying \({4}\) and \({2}\) to fill the left box on the top row.
- \({4}\times{2}={8}\)
- Write \({0}\) and \({8}\) to show that there are no tens.
- Now multiply \({3}\) and \({2}\) to fill the right box on the top row.
- \({3}\times{2}={6}\)
- Next, multiply \({4}\) and \({6}\) to fill the left box on the bottom row.
- \({4}\times{6}={24}\)
- Remember to put the \({2}\) above the diagonal.
- Complete the grid by multiplying \({3}\) and \({6}\) to fill the right box on the bottom row.
- \({3}\times{6}={18}\)
After completing the grid, add the columns along the diagonals, starting at the bottom-right.
We sometimes need to carry over from one diagonal to the next.
To get the answer, read the totals down the left and to the right.
\({43}\times{26}={1,118}\)
Question
Use Napier’s method to calculate \({264}\times{53}\).Draw a \({3}\times{2}\) box and follow the guidelines in the previous example.
Answer
\({264}\times{53}={13,992}\)
Multiplication - area method
The area method breaks down the numbers to be multiplied at the sides of a rectangle.
The area of the rectangle will be the answer to the multiplication.
Example
Calculate:
\({56}\times{34}\)
Therefore:
\(({50}+{6})\times({30}+{4})\)
To calculate the answer, the individual areas need to be added together:
\({56}\times{34}={1,500}+{180}+{200}+{24}={1,904}\)
Question
Use the area method to calculate:
\({236}\times{27}\)
Answer
\({236}\times{27}\)
Therefore \(({200}+{30}+{6})\times({20}+{7})\)
\({236}\times{27}={4,000}+{600}+{120}+{1,400}+{210}+{42}={6,372}\)
Short multiplication
To multiply \(237\) by \(4\) without using a calculator, you can set it out like this:
- Start with \(4 \times 7\), which is \(28\), so write the \(8\) and carry the \(2\) to the tens column.
- \(4 \times 3 = 12\), but remember to add the carried \(2\) to get \(14\). Write the \(4\) and carry the \(1\) to the hundreds column.
- \(4 \times 2 = 8\), and we add the carried \(1\) to get \(9\).
Therefore:
\(237 \times 4 = 948\)
This method is called short multiplication.
Short division
To divide a large number by a \({1}\)-digit number you can set it out like this:
\(6\) goes into \(9\) once with \(3\) remaining (remainder \(3\)), so put \(1\) above the hundreds column and carry the \(3\) to the 1.
\(6\) goes into \(31\) five times, remainder \(1\), so put \(5\) above the tens column and carry the \(1\) to the units column.
\(6\) goes into \(18\) exactly three times, so put \(3\) above the units column.
Read the answer from the top line, \({153}\).
Sometimes, a number does not divide into another number exactly.
In this case, we can either give the answer as a whole number along with a remainder, or we can give the answer as a decimal.
The decimal answer may be a or a .
Often, decimal answers may be rounded to a required number of .
For example:\({964}\div{7}\)
Method 1 - answer as a whole number with a remainder
- \(7\) goes into \(9\) once with \(2\) remaining (remainder \(2\)), so put \(1\) above the hundreds column and carry the \(2\) to the tens column.
- \(7\) goes into \(26\) three times, remainder \(5\), so put \(3\) above the tens column and carry the \(5\) to the units column.
- \(7\) goes into \(54\) seven times, remainder \(5\), so put \(7\) above the units column and have a remainder of \(5\).
So, \(964 \div 7 = 137\) remainder \(5\).
We write this with an \(r\) for 'remainder', so it looks like this:
\({964}\div{7}={137~r5}\)
Method 2 - decimal answer, rounded to decimal place
- \(7\) goes into \(9\) once with \(2\) remaining (remainder \(2\)), so put \(1\) above the hundreds column and carry the \(2\) to the tens column.
- \(7\) goes into \(26\) three times, remainder \(5\), so put \(3\) above the tens column and carry the \(5\) to the units column.
- \(7\) goes into \(54\) seven times, remainder \(5\), so put \(7\) above the units column and carry the \(5\) to the tenths column (writing the \({964}\) as \({964.000}\) as \({7}\) does not divide into it exactly).
- \({7}\) goes into \({50}\) seven times, remainder \({1}\), so put \({7}\) above the tenths column and carry the \({1}\) to the hundredths column.
- \({7}\) goes into \({10}\) once, remainder \({3}\), etc.
So, \({964}\div{7}={137.714…}\)\({=137.7}\) (correct to \({1}\) decimal place).
This method is called short division.
Multiplying and dividing by numbers between 0 and 1
Multiplying by a number between 0 and 1
The multiplication sign can be replaced by the phrase 'lots of'.
For example:
\(2 \times 3\) means \(2\) lots of \(3\) (\({=6}\))
\(6 \times 8\) means \(6\) lots of \(8\) (\({=48}\))
So, \({10}\times\frac{1}{2}\) means \({10}\) lots of \(\frac{1}{2}\) (\({=5}\))
And \({12}\times\frac{1}{3}\) means \({12}\) lots of \(\frac{1}{3}\) (\({=4}\))
When you multiply by a number greater than 1, the answer is greater than the original number.
But when you multiply by a number between 0 and 1, the answer is smaller than the original number.
We have seen that: \({10}\times\frac{1}{2}={5}\)
We also know that \({10}\div{2}={5}\)
Therefore: \({10}\times\frac{1}{2}={10}\div{2}\)
This shows that '\(\times~\frac{1}{2}\)' is the same as '\(\div~{2}\)'.
In general:
\(m \times \frac{1}{n} = m \div n\)
Examples
\(8 \times \frac{1}{4} = 8 \div 4 = 2\) (as '\(\times~\frac{1}{4}\)' is the same as '\(\div~{4}\)')
\(20 \times \frac{1}{5} = 20 \div 5 = 4\) (as '\(\times~\frac{1}{5}\)' is the same as '\(\div~{5}\)')
Dividing by a number between 0 and 1
Imagine that you had \(10\) bars of chocolate that you wanted to share amongst some children.
If you gave the children \(2\) bars each, you would have enough for \(5\) children.
\(10 \div 2 = 5\)
If you gave the children \(\frac{1}{2}\) bar each, you would have enough for \(20\) children.
\(10 \div \frac{1}{2} = 20\)
The pattern
Can you see what's happening?
\(10 \div 2 = 5\)
\(10 \div \frac{1}{2} = 20\)
When you divide by a whole number the answer is smaller than the original number.
When you divide by \(\frac{1}{2}\) the answer (\({20}\)) is greater than the original number (\({10}\)).
When you divide by a number greater than 1, the answer is smaller than the original number. But when you divide by a number between 0 and 1, the answer is greater than the original number.
So: \(10 \div \frac{1}{2} = 20\)
Similarly: \(10 \div \frac{1}{3} = 30\) and \(10 \div \frac{1}{4} = 40\)
In general: \(m \div \frac{1}{n} = mn\)
Questions
Q1. What is \(10 \div \frac{1}{7}\)?
Q2. Find the value of \(4 \div \frac{1}{3}\)
Answers
A1. \({10}\div\frac{1}{7}={10}\times{7}={70}\)
A2. \(4 \div \frac{1}{3} = 4 \times 3 = 12\)
The great chocolate challenge: Multiplication and division
Yeah, yeah… No, no it’s all finished! 40 lovely brownies, yeah.
They smell lovely. Mmm.
I’ll be at the cake stall 8am tomorrow, don’t worry.
I’ll bring my 40 lovely brownies. OK.
I should have done this earlier.
This recipe is for 12 brownies and I need at least 40.
If I use multiplication and multiply all the ingredients by four I’ll get 12 x 4 which is 48 brownies and I keep eight for myself. Great!
So, flour to start.
I’ve got 500 grams and the recipe says 100g of plain flour for 12.
I can do this kind of multiplication in my head.
4 x 100 is 400g.
Now, chocolate, 350g for 12 brownies.
So I multiply by four.
That’s 350 x 4 - that should still be possible in my head.
One way of multiplying by four is to break it down and multiply it by two and then multiply it by two again.
So I double 350, that comes to 700 and then I double it again, comes to 1400.
Oh no, I had seven bars at 200g per bar.
That would have given me 1400g exactly. I’ve only gone and eaten half a bar!
Oh well, I’ll put in the six bars so that’s 1200g and then I’ll have to do the maths properly.
So let’s get all the ingredients together.
Let’s slow it down and get to grips with the basic idea.
I promised the cake stall that I’d make 40.
So I have to work out the quantity of the ingredients that I need to make 40 brownies.
So imagine I made 12 brownies as my recipe. You can almost smell them.
First of all I need to divide by 12 to find out the ingredients for one brownie.
Then I need to multiply by 40 to find out the ingredients for 40.
First I’ll do the chocolate.
I’ll divide 350 by 12 and then multiply it by 40.
Now dividing 350 by 12?
I think I’ll just use a calculator.
Oh come on! Where are you?
Oh no! My little sister, again!
So how am I going to work this out?
Oh that’s a lot of maths!
Basically, I’m just doing the same thing every time.
I’m dividing by 12 to work out the ingredients for one and then multiplying by 40 to work out the ingredients for 40.
It’d really speed things up if I could work out a single amount to multiply all the ingredients by.
Oh and I’ve got lots of this!
So, what do I have to multiply the ingredients of 12 brownies by to make 40 brownies?
Well I already worked out that four times the quantities would give me 48 and that’s too much because of the chocolate shortage.
And three times the quantities would give me enough for 36 brownies.
Which isn’t enough brownies.
So the answer I’m looking for lies between three and four.
Hmm, what’s that number?
I also know that the opposite of multiplying is dividing.
So to find the value that I’m looking for I need to divide 40 by 12.
Now, how do I do this?
I could use long division but it’s quite a small number for that.
Let’s just break it down.
I know that 3 x 12 is 36.
So 12 goes into 40, three times remainder four.
So I have to divide the reminder by 12.
I can show this as a fraction. Four divided by 12.
I can simplify the fraction.
Both the numerator and denominator are divisible by four.
If I divide both by four I get one over three, which is a third.
So adding that back on to the three.
The value I’m looking for is three and a third.
So starting with the chocolate.
Hey! I can use the calculator on my phone!
Three and a third, that’s 3.33333333 times the 350g of chocolate.
So I need exactly 1,166.6 recurring grams of chocolate.
So having put in 1200g I’ve got to eat about 30g of it.
Someone’s got to do it.
Test section
Question 1
Calculate \({46}\times{8}\)
Answer
The correct answer is \({368}\).
Question 2
Calculate \({145}\times{7}\)
Answer
The correct answer is \({1,015}\).
Question 3
Use any appropriate method to calculate \({34}\times{24}\)
Answer
The correct answer is \({816}\).
Question 4
Use any appropriate method to calculate \({653}\times{15}\)
Answer
The correct answer is \({9,795}\).
Question 5
Calculate \({95}\div{5}\)
Answer
The correct answer is \({19}\) exactly.
Question 6
Calculate \({213}\div{3}\)
Answer
The correct answer is \({71}\) exactly.
Question 7
Calculate \({307}\div{4}\), giving your answer as a decimal.
Answer
The correct answer is \({76.75}\).
Question 8
Calculate \({564}\div{8}\), giving your answer as a decimal.
Answer
The correct answer is \({70.5}\).
Question 9
\({5}\) buses carry \({49}\) people each.
What are the total number of people on the \({5}\) buses?
Answer
The correct answer is \({245}\).
Question 10
An egg box holds \({6}\) eggs.
If there are \({165}\) eggs, how many boxes will be full and how many eggs will be remaining?
Answer
The correct answer is \({27}\) boxes and \({3}\) eggs remaining.
More on Operations/calculations
Find out more by working through a topic
- count4 of 6
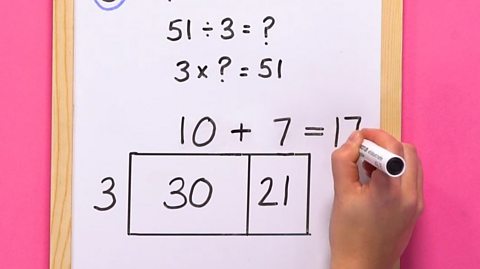
- count5 of 6
